This Page contains information about an experiment done with a single photon counting CCD camera. A brief description of the experiment is given and then the results are shown. The experiments (as well as a number of others) were carried out under an NSF ILI grant 9251313 to Robert Austin and Lyman Page at Princeton University.

Movie added at the end. Please feel free to use. -L.Page. Posted July 5, 2010

Background

• Our classical notions of what constitutes waves and particles are hopeless when it comes to interpreting quantum phenomena. Perhaps the most lucid explanation of the peculiar manner in which quanta act is given in the first chapter of the third volume of the Feynman lecture series. Although Feynman talks about electrons acting as both particles and waves, we may apply the same reasoning to photons. Most students of physics learn about Young's double slit diffraction in their first year. In the limit of very thin slits separated by a distance d, the intensity pattern on a screen a distance D$>>$d behind the slits is given by:

• $$ I = I_0\cos^{2}\bigr({2\pi d\over 2\lambda}\sin(\theta )\bigl) $$

• where $\theta$ is the angle from the center of the slits to the point on the screen where the intensity is measured. The essential point is that the waves are spread over a range in angles and there are multiple maxima (many more than the number of slits). Light may also be considered from the quantum point of view. Photomultiplier tubes count single photons; many texts show the positron/electron pairs from the decay of a gamma ray. If we think of the photons as rocks then if they are thrown at a wall containing two holes they should land in two piles on the other side of the wall. In the demonstration, we illuminate a triple slit with a laser beam that has been attenuated to such a degree that the {\it mean} path between photons is $\approx 2\times 10^{3}$ meters. We show that despite this large distance, a diffraction patterns obtains -- the photon has ``interfered'' with itself.

• The ability to demonstrate this phenomena has been around for a long time, G. Reynolds did it in 1938 and a Soviet group did it (incorrectly) before him. With the advent of photon counting CCD cameras the demonstration is easy and visually startling.

  Phil Nelson (U. Penn) shared an article with us from 1909. In a paper entitled ``Interference Fringes with Feeble Light,'' G. I. Taylor shows that a 2000 hour exposure of highly attenuated X-rays (Rontgen rays) still produces a diffraction pattern. The paper is reprinted in ``The Pleasures of Counting'' by T. Koerner. (added LP Feb 2011)

The Demonstration

• A 40 by 80 cm aluminum box of depth 25 cm holds the slits and a mirror. The inside of the box is painted flat black and black felt is used near all the seams. A 1 mW He-Ne laser ($\lambda = 632.8 {\rm nm}$, $3\times 10^{15}~$photons/sec) illuminates a 1/8 diameter hole drilled into one end of the box. Neutral density filters mounted in a black felt-lined holder attenuate the laser light by a factor of $\alpha = 5\times10^{-11}$. Thus, the mean distance between photons is 2 km. Then, the attenuated light passes through the slits, bounces off the mirror at the far end of the box and returns to enter the aperture of a Hamamatsu model C2400-30 photon counting camera. The top of the aluminum box is hinged for easy access and all the alignment is done with the neutral density filters removed.

• The Hamamatsu camera is a remarkable device. In essence, it has two successive micro-channel plates followed by a CCD chip. The camera has a 768 by 420 pixel array that is read at video rates. At maximum sensitivity, near $\lambda = 400~$nm, the camera's quantum efficiency is near .3; near $\lambda = 680~$nm, it is 0.001. The images are acquired with an Argus-10 video receiver also made by Hamamatsu. The Argus can average many video frames and displays the data on a video screen. The output of the Argus is fed to CCD frame-grabber card (we use a Dipex card but most any will do) controlled by a 486DX based computer. The computer periodically samples the video output of the Argus and stores the data for future use.

• The following pictures were taken with the above setup. The width of each slit is 0.04 mm and the separation is 0.125 mm. The coherence length of the laser has not yet been measured.

This shows the result after counting for 1/30 second. The few white dots in the image are photons. One expects to get $3.2\times10^{15}$ X $\alpha$ X (quantum efficiency) X (time) = 5 photons. Note that the photons are distinct.

This is the image after averaging for 1 second. The interference pattern is beginning to immerge but it is clear that it is made up of photons. Though one might believe that there are three ``piles of rocks,'' one would be hard pressed to be sure that there were more.

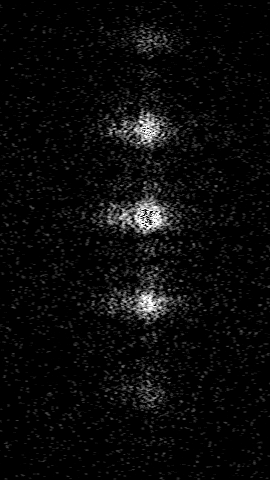
Same image as the above after averaging for 100 seconds. Even though there were only three slits, 5 maxima are clearly visible. Here we have an interference pattern made up of discrete particle detections.

Ye Ma and Omelan Stryzak put together a movie of the photon counting some time ago. In this particular instance, the light level is pretty high. The movie starts out after some accumulation (integration) has occurred. In the middle of the movie, the accumulation is reset and one can see the raw input of single counts. The pattern then builds up again.

Movie of counting

References

• The Feynman Lectures on Physics. Vol. 3, R. P. Feynman, R. B. Leighton, & M. Sands, Addison-Wesley, 1965

Lyman Page / [email protected]